Matrix Puzzle
Time Limit: 8000/4000 MS (Java/Others) Memory Limit: 32768/32768 K (Java/Others)Total Submission(s): 65 Accepted Submission(s): 0
Problem Description
As you know, math is quite incredible.For example,if you want to know all x that not only satisfy the expression A^x = B ( mod C) where A,B and C are given and C is a prime number, but also smaller than C, you could use the following algorithm
(http://en.wikipedia.org/wiki/Baby-step_giant-step).
Now your task is quite simple. You are given A,B, here A and B are matrixes( n X n).You are expected to solve the following expression.
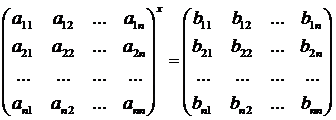 mod 1000000007
mod 1000000007
(http://en.wikipedia.org/wiki/Baby-step_giant-step).
Now your task is quite simple. You are given A,B, here A and B are matrixes( n X n).You are expected to solve the following expression.
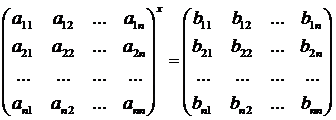 mod 1000000007
mod 1000000007Input
There are no more than 20 cases.
For each case,there are two integers n,L in the first line indicating the size of the matrixes , the value of L. (Here 1<= n <= 50, 1<= L <= 10^11)
Then follows 2n lines.
For the first n lines, indicating the matrix A.
For the next n lines, indicating the matrix B.
All the numbers in the matrix are between 0 and 10^9
For each case,there are two integers n,L in the first line indicating the size of the matrixes , the value of L. (Here 1<= n <= 50, 1<= L <= 10^11)
Then follows 2n lines.
For the first n lines, indicating the matrix A.
For the next n lines, indicating the matrix B.
All the numbers in the matrix are between 0 and 10^9
Output
Output a single line with “Case #idx: ”,here idx is the case number start from 1. Then output if there is only one valid solution (the solution must no larger than L and larger than 0).
If there is only one valid solution ,then just output “Yes”, otherwise output “No”.
We assure that the ABS(|A|) (determinant’s abosolute value)is always larger than one after the mod operation in all the cases.
If there is only one valid solution ,then just output “Yes”, otherwise output “No”.
We assure that the ABS(|A|) (determinant’s abosolute value)is always larger than one after the mod operation in all the cases.
Sample Input
1 6 2 2 1 4 2 1 2 3 1 2 2 1 5 4 4 5
Sample Output
Case 1: Yes Case 2: No Case 3: Yes
Author
AekdyCoin@FZU
