
| Online Judge | Online Exercise | Online Teaching | Online Contests | Exercise Author |
|
F.A.Q Hand In Hand Online Acmers |
Best Coder beta VIP | STD Contests DIY | Web-DIY beta |
Pocket Cube
Time Limit: 20000/10000 MS (Java/Others) Memory Limit: 32768/32768 K (Java/Others)Total Submission(s): 3140 Accepted Submission(s): 906
Problem Description
Pocket Cube is a 3-D combination puzzle. It is a 2 × 2 × 2 cube, which means it is constructed by 8 mini-cubes. For a combination of 2 × 2 mini-cubes which sharing a whole cube face, you can twist it 90 degrees in clockwise or counterclockwise direction, this twist operation is called one twist step.
Considering all faces of mini-cubes, there will be totally 24 faces painted in 6 different colors (Indexed from 0), and there will be exactly 4 faces painted in each kind of color. If 4 mini-cubes' faces of same color rely on same large cube face, we can call the large cube face as a completed face.

Now giving you an color arrangement of all 24 faces from a scrambled Pocket Cube, please tell us the maximum possible number of completed faces in no more than N twist steps.
Index of each face is shown as below:
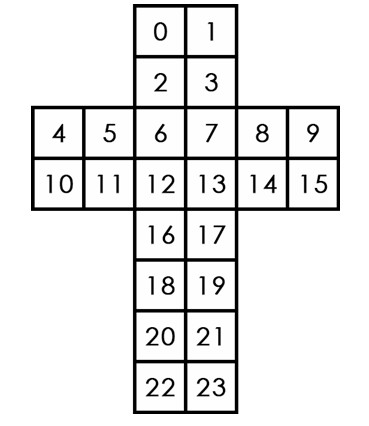
Considering all faces of mini-cubes, there will be totally 24 faces painted in 6 different colors (Indexed from 0), and there will be exactly 4 faces painted in each kind of color. If 4 mini-cubes' faces of same color rely on same large cube face, we can call the large cube face as a completed face.

Now giving you an color arrangement of all 24 faces from a scrambled Pocket Cube, please tell us the maximum possible number of completed faces in no more than N twist steps.
Index of each face is shown as below:

Input
There will be several test cases. In each test case, there will be 2 lines. One integer N (1 ≤ N ≤ 7) in the first line, then 24 integers Ci separated by a single space in the second line. For index 0 ≤ i < 24, Ci is color of the corresponding face. We guarantee that the color arrangement is a valid state which can be achieved by doing a finite number of twist steps from an initial cube whose all 6 large cube faces are completed faces.
Output
For each test case, please output the maximum number of completed faces during no more than N twist step(s).
Sample Input
1 0 0 0 0 1 1 2 2 3 3 1 1 2 2 3 3 4 4 4 4 5 5 5 5 1 0 4 0 4 1 1 2 5 3 3 1 1 2 5 3 3 4 0 4 0 5 2 5 2
Sample Output
6 2
Source
| Home | Top |
Hangzhou Dianzi University Online Judge 3.0 Copyright © 2005-2025 HDU ACM Team. All Rights Reserved. Designer & Developer : Wang Rongtao LinLe GaoJie GanLu Total 0.000000(s) query 1, Server time : 2025-03-29 20:35:49, Gzip enabled |
Administration |