Minimum Heap

Alex is curious about data structures. He is working on binary trees recently and particularly interested in complete binary trees.


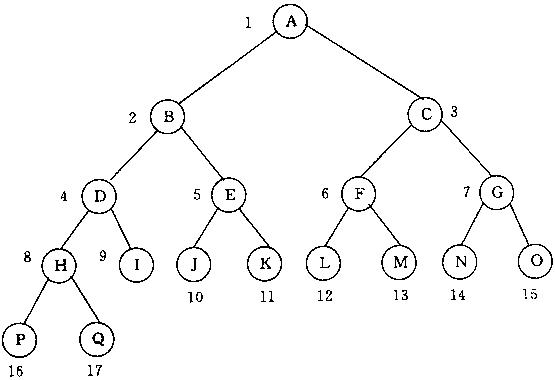
A complete binary tree satisfies that all levels of the tree, except possibly the last one (deepest) are fully filled, and, if the last level of the tree is not complete, the nodes of that level are filled from left to right.
Alex defines his own complete binary tree: each node has a weight, while father's is always less than or equal to its sons'. He names this complete binary tree as minimum heap.

Now he wants to know: With N nodes weighted from 1 to N (each appears once), how many heaps can be created. The answer (represented by Q) may be very large, so please output a number P while P = Q mod M.

The input consists of several test cases. The first line contains a number T, indicating the number of test cases. Each test case is on a single line, and it consists the number N and M.
Technical Specification
1. 1 ≤ T ≤ 10
2. 1 ≤ N ≤ 1000
3. 2 ≤ M ≤ 1000,000,000

For each test case, output the number P on a single line.

2
1 9973
100 9973

1
174

The 4th Baidu Cup final