
| Online Judge | Online Exercise | Online Teaching | Online Contests | Exercise Author |
|
F.A.Q Hand In Hand Online Acmers |
Best Coder beta VIP | STD Contests DIY | Web-DIY beta |
AVL Tree
Time Limit: 1000/1000 MS (Java/Others) Memory Limit: 32768/32768 K (Java/Others)Total Submission(s): 1416 Accepted Submission(s): 631
Problem Description
An AVL tree is a kind of balanced binary search tree. Named after their inventors, Adelson-Velskii and Landis, they were the first dynamically balanced trees to be proposed. Like red-black trees, they are not perfectly balanced, but pairs of sub-trees differ in height by at most 1, maintaining an O(logn) search time. Addition and deletion operations also take O(logn) time.
Definition of an AVL tree
An AVL tree is a binary search tree which has the following properties:
1. The sub-trees of every node differ in height by at most one.
2. Every sub-tree is an AVL tree.
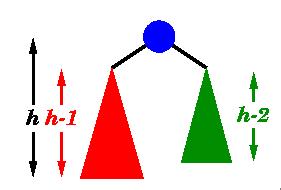
Balance requirement for an AVL tree: the left and right sub-trees differ by at most 1 in height.An AVL tree of n nodes can have different height.
For example, n = 7:
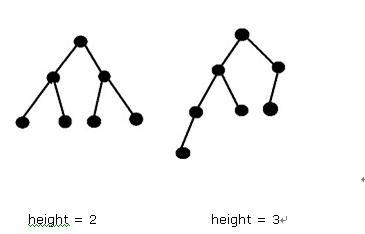
So the maximal height of the AVL Tree with 7 nodes is 3.
Given n,the number of vertices, you are to calculate the maximal hight of the AVL tree with n nodes.
Definition of an AVL tree
An AVL tree is a binary search tree which has the following properties:
1. The sub-trees of every node differ in height by at most one.
2. Every sub-tree is an AVL tree.

Balance requirement for an AVL tree: the left and right sub-trees differ by at most 1 in height.An AVL tree of n nodes can have different height.
For example, n = 7:

So the maximal height of the AVL Tree with 7 nodes is 3.
Given n,the number of vertices, you are to calculate the maximal hight of the AVL tree with n nodes.
Input
Input file contains multiple test cases. Each line of the input is an integer n(0<n<=10^9).
A line with a zero ends the input.
A line with a zero ends the input.
Output
An integer each line representing the maximal height of the AVL tree with n nodes.
Sample Input
1 2 0
Sample Output
0 1
Author
scnu
| Home | Top |
Hangzhou Dianzi University Online Judge 3.0 Copyright © 2005-2024 HDU ACM Team. All Rights Reserved. Designer & Developer : Wang Rongtao LinLe GaoJie GanLu Total 0.000000(s) query 1, Server time : 2024-11-22 09:34:02, Gzip enabled |
Administration |